Acid-base titration
Tutorial 15
pH during titration of a weak acid by a strong base
Schematic:
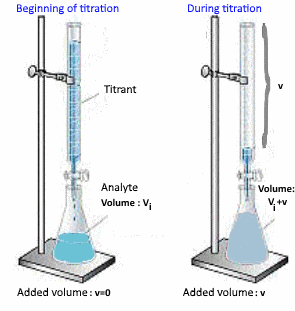
Determining the base volume $ V_e $ added at the equivalent point
$ n_{added \;base } = n_{initial \; acid} $
$ V_e \cdot c_{base} = V_i \cdot c_{acid} $
Hence $ V_e $
pH by volume $ v $ of base added
$ v = 0 $
pH of a weak acid of molarity $ c_{acid} $:
$ x = [H_3O^+] $
$ x ^ 2 + c_{acid} x-c_{acid} K_a = 0 $
Etc.
$ v \lt V_e $
Determine the number of moles of acid $ n_a $ which have not yet reacted as well as the number of moles of weak base $ n_b $ formed.
pH of buffer
$ pH = pK_a + log\frac{n_b}{n_a} $
$ v = V_e $
pH of a weak base, molarity: $ c_b $:
The number of moles of weak base at this time $ n_b $ $ = $ $ n_{added \; base} $ $ = $ $ n_{initial \; acid} $
Then: $ c_b = \frac{n_b}{V_i + V_e} $
Then
$ x = [OH^-] $:
$ x^2 + c_bx-c_bK_b = 0 $
etc...
$ v \gt V_e $
Determine the numbers of strong base moles $ n_b $ in excess
$ pH = 14 + log \frac{n_b}{V_i + v} $
$ 20 \; mL \; HCOOH \; 0.10 \; M $ are titrated by $ NaOH \; 0.1 \; M $. Calculate the pH at the beginning of the titration.
$ HCOOH $ is what kind of an acid ?
For answers, use (possibly several times) the arrows ↑ Down! and ↓ Up!
Complete please this question before moving on to the next one!
Calculate $ [H_3O^+] $ by a second degree equation!
Let $x$ $=$ $H_3O^+$
$x^2+c_ax-c_aK_a$ $=$ $0$
$x^2+0.10\cdot x-0.10\cdot 10^{3.75}$ $=$ $0$
$x=4.27\cdot 10^{-3}\;M$
$pH$ $=$ $-log[H_3O^+]$ $=$ $-log(4.27\cdot 10^{-3})$ $=$ $2.37$
