Use arrows ↓ and ↑ !
The electromotive force (→ potential) under the standard conditions of the folowing next battery is worth $0.45 \;V$:
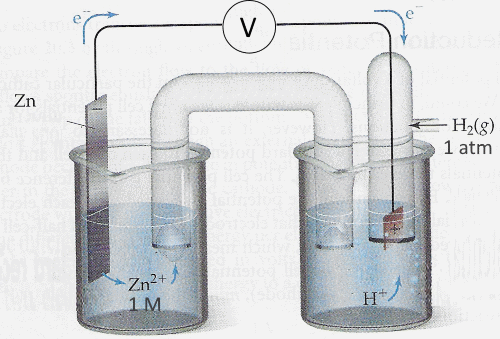Calculate $[H^+]$ !
$E^o_{battery}$ $ =$ $ 0-(-0.76)=0.76\; V$
$n\; =\; 2$ indeed $2H^+$ + 2$e^-$ + $Zn$ - 2$e^- $ $\leftrightarrows$ $Zn^{2+}+H_2$
$E$ $ =$ $ E^o_{battery}-\frac{0.059}{2}log\frac{[Zn^{2+}][P_{H_2}]}{ [H^+]^2}$ $0.45$ = $ 0.76-\frac{0.059}{2}log\frac{1.0\cdot 1.0}{[H^+]^2} $ $[H^+]$ =$\sqrt{3.3\cdot 10^{-11}}$ = $5.7\cdot 10^{-6} M$