Acid-base titration
Tutorial 11



 pH during titration of a strong acid by a strong base
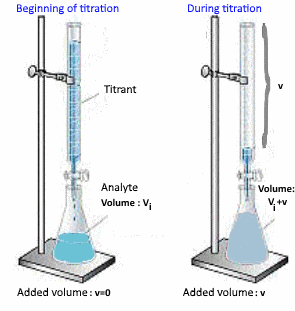
Schematic:
pH during titration of a strong acid by a strong base
Schematic:
Determination of the base volume $ V_e $ added at the equivalent point
$n_{added\;base}$ $= $ $n_{initial\;acid}$
$V_e\cdot c_{base}$ $=$ $V_i\cdot c_{acid}$
so $V_e$ is calculated.
Calculation of pH ($v$ = volume of added base)
$v=0$
pH of a strong acid with molarity $c_{acid}$:
$pH=-log\;c_{acid}$
$v\lt V_e$
Determine the number of moles of acid $ n_a $ that have not yet reacted, then:
$pH$ $=$ $-log\frac{n_a}{V_i+v}$
$v=V_e$
$pH=7$
$v\gt V_e$
Determine the numbers of base moles $ n_b $ in excess, then:
$pH$ $=$ $14+log\frac{n_b}{V_i+v} $
$ 0,183 \; g \; HCl$ are dissolved in water forming $50\; ml$ of solution.
$ 10 \; mL $ of this solution are titrated by $ NaOH \; 0.050 \; M $.
Calculate the pH after adding $ 20 \; mL \; NaOH $.
Calculate first the initial molarity of $ HCl $ !
For answers, use (possibly several times) the arrows ↑ Down! and ↓ Up!
Complete please this question before moving on to the next one!
$c_{HCl}$ $=$ $\frac{0.183}{36.5\cdot 0.050}$ $=$ $0.10\;M$
Calculate the volume added at the E.P.!.
$V_e$ $=$ $\frac{0.010\cdot 0.10}{0.050}$ $=$ $20\;mL$
We are at the E.P., so:
$pH=7.0$



