

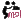

A sample of a carbon, oxygen, and hydrogen compound has a mass of $ 50\;g $. Its molar mass is worth $ 60 \frac{g}{mol} $. By burning it, we have isolated $ 73.3\;g \;CO_2 $ and $ 29.7 \;g \; H_2O $. Note that the carbon and hydrogen of these products must originate integrally from the substance, but not the oxygen some of which has been used to burn the substance! First calculate the mass of $ H $ and $ C $ and then that of $ O $ in the sample and then in one mole of that substance!
For answers, use (possibly several times) the arrows ↑ Down! and ↓ Up! Complete please this question before moving on to the next one!
In the sample: $44\;g \; CO_2$ contain $12\;g \; C$ donc $73,3\;g \; CO_2$ contain $\frac{12\cdot 73.3}{44}$ $=$ $20,0\;g\;C$ which was in $ 50\;g $ of substance. $18\;g \; H_2O$ contain $2\;g \; H$ donc $29,7\;g \; H_2O$ contain $\frac{2\cdot 29.7}{18}$ $=$ $3.33\;g\;H$ which was in $ 50\;g $ of substance. In one mole: $m_C$ $=$ $\frac{20\cdot 60}{50}$ $=$ $24.0\;g\;C$ $m_H$ $=$ $\frac{3,33\cdot 60}{50}$ $=$ $4.0\;g\;H$ $m_O$ $=$ $60-4-24$ $=$ $32\;g\;O$
Transform these masses into moles of $C$,$H$ and $O$!
$n_{C}= \frac{m_{C}}{M_C}$ $=$ $\frac{24}{12}$ $=$ $2\;mole\; C$ $n_{H}= \frac{m_{H}}{M_H}$ $=$ $\frac{4,0}{1,0}$ $=$ $4\;mole\; H$ $n_{O}= \frac{m_{O}}{M_O}$ $=$ $\frac{32}{16}$ $=$ $2\;mole\; O$
Formula? Formule: $C_2H_4O_2$