





Suppose we have a gas bubble surrounded by a thin flexible membrane and maintained at a constant temperature in the liquid:

Case 1: Initially, the pressure inside the bubble is below the pressure inside the liquid (ambient pressure):

The bubble will contract reaching finally (Boyle and Mariotte Law: Volume decreases, so pressure increases!) the pressure of the liquid.
Case 2: Initially, the pressure inside the bubble is higher than the pressure inside the liquid (ambient pressure):
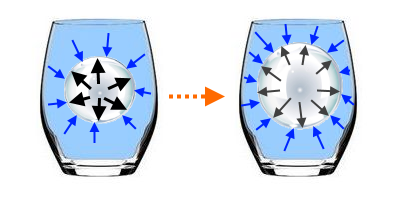
The bubble will expand reaching finally (Boyle and Mariotte Law: Volume increases, so pressure decreases!) the pressure of the liquid.
Suppose a vapor bubble is in its liquid ( one substance) without partition membrane:
Case 1: $P_o\;\lt\;P$ (*) At first, the saturation vapor pressure of the bubble is below the liquid pressure (ambient pressure):
The bubble will contract trying to have a pressure equal that of the liquid. But saturation vapor pressure is maintained (because neither the temperature nor the nature of the substance do change) and we fall back to condition (*) The contraction will continue until all the vapour will be condensed!

$P_o\;\lt\;P$ $\longrightarrow$ bubbles disappear by condensation!
Case 2: $P_o\;\gt\;P$ (**) At first, the saturation vapor pressure of the bubble is higher than the liquid pressure (ambient pressure):
The bubble will expand trying to have a pressure equal that of the liquid. But saturation vapor pressure is maintained (because neither the temperature nor the nature of the substance do change) and we fall back to condition (**) The expansion will continue and lead to the boiling of the liquid!

$P_o\;\gt\;P$ $\longrightarrow$ proliferation of bubbles by boiling !
We have just seen that - by raising the temperature of a liquid substance we increase its saturation vapor pressure. - the boiling of a liquid substance should commence when the ambient pressure is equal to its saturation vapor pressure.
The boiling point of a substance is the temperature at which the saturated vapor pressure is equal to the ambient pressure !

The diagram shows the saturation vapour pressures of three liquids in function of temperature: 1 Ether 2 Ethanol 3 Water The boiling temperatures at ambient pressure $101.3\;kPa$ (sea level) are determined (blue dots). It can still be seen that the higher the saturated vapor pressure at a given temperature, eg room temperature (green dots) plus the boiling point is low (orange dots)
The higher the saturated vapor pressure at a given temperature of a substance the lower will be its boiling point.
Exercise Determine on the diagram above the boiling temperatures of the three liquids 1. on Mount Everest $(P = 253\;torr)$ 2. on Mont Blanc $(P = 0.54\;atm)$