





Thereafter we call $x$ the volume $(mL)$ of $NaOH$ already added.
$c_{Α}$ = $\frac{c_BV_B}{V_{Α}}$ = $\frac{0.05\cdot 0.040}{0.020}$ = $0.10 \frac{mol}{L}$
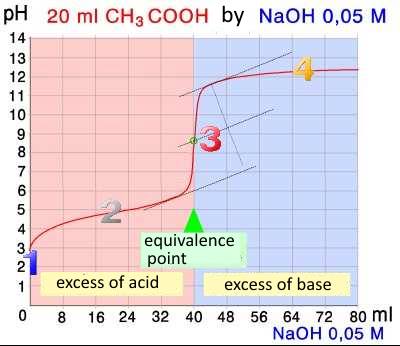
1.
The weak acid $CH_3COOH$ is present alone. its concentration is $c_{Α}=0.10 \;M$ Weak acid! $pH$ $=$ $\frac{1}{2}pK_a$ $-$ $\frac{1}{2}logc_{Α}$ = $\frac{1}{2}4.75$ $-$ $\frac{1}{2}log0.1$ = $2.88$
2.
(For simplicity. we consider before the substances first as if they were not dissociated)
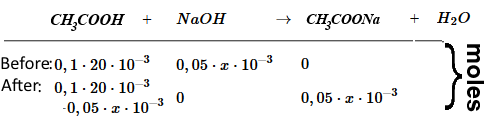
Buffer! $pH$ = $pK_a$ $+$ $log( \frac{n_{CH_3COONa}}{n_{CH_3COOH}})$ $4.75$ $+$ $log( \frac{0.05\cdot x\cdot10^{-3}}{0.1\cdot20\cdot 10^{-3}-0.05\cdot x\cdot10^{-3}})$ $pH$ $=$ $4.75$ $+$ $log( \frac{0.05\cdot x\cdot}{0.1\cdot20-0.05\cdot x})$
3.
$CH_3COOH$ and $NaOH$ reacted completely. remains a solution of the weak base $CH_3COO^-$: $pH$ = $ 14$ $ -$ $\frac{1}{2}pK_B$ $+$ $\frac{1}{2}log (c_{CH_3COONa})$ = $14$ $-$ $\frac{1}{2}9.25$ $+$ $\frac{1}{2}$ $\cdot$ $log (\frac{0.1\cdot20\cdot 10^{-3}}{(20+40)\cdot 10^{-3}})$ = $8.63$
4.

Weak base neglected! Strong base! $c_{NaOH}$ = $\frac{n_{NaOH}}{(V_{Α}+x)10^{-3}}$ = $\frac{0.05\cdot x\cdot10^{-3}-0.1\cdot20\cdot10^{-3}}{(20+x)10^{-3}}$ = $\frac{0.05\cdot x-2}{20+x}$ $pH$ $=$ $14+log( \frac{0.05\cdot x-2}{20+x})$
→ Here you find simulations of such titrations