





Two test tubes contain different quantities of iodine . We have a solid phase where iodine is pure and a gas phase. The system is heterogeneous. The two tubes are put at the same temperature:
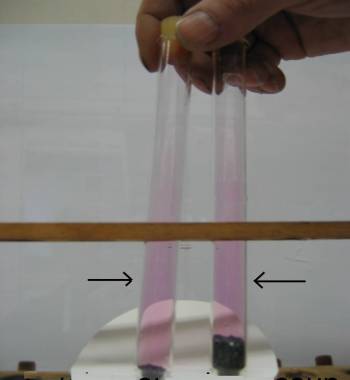
The intensity of coloration in the gas phase (therefore the partial pressure of iodine) seams to be the same.
As iodine is alone in the lower solid phase, its concentration $c=[I_2(s)]$ is constant therein. We apply the law of mass action: $\frac{[I_2(g)]}{[I_2(s)]}$ $=$ $K$ $\frac{[I_2(g)]}{c}$ $=$ $K$ $[I_2(g)]$ = $K\cdot c $ Using the ideal gas law, let us examine the partial pressures of iodine in the gas phase : $P_{I_2(g)}$ $= $ $[I_2(g)]\cdot R\cdot T$ $P_{I_2(g)}$ $= $ $K \cdot c \cdot R\cdot T$ A new constant is found as a product of four constants: $P_{I_2(g)}$ $= $ $K_{sat}$
When a substance $X$ is in equilibrium between gaseous phase and a pure liquid or solid phase , and when it stays pure in the last phase, its partial pressure in the gaseous phase is constant at a given temperature when equilibrium is attained: $P_X(g)$ $=$ $K_{sat}$ $K_{sat}$ is a constant at a given temperature called saturation vapour pressure of substance X in the gaseous phase.