Untersuchung des pH-Wertes während einer Titration einer schwachen Säure durch eine starke Base:

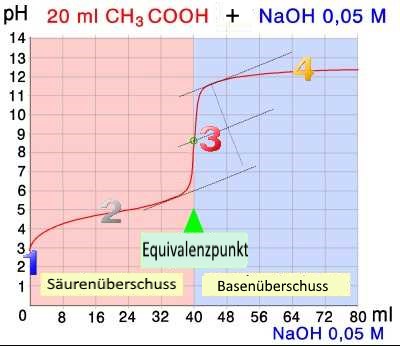
Anschließend bezeichnen wir durch $ x $ das Volumen (in $ mL $) von bereits hinzugefügtem $ NaOH $ .

Anfangskonzentration der Säure

$c_{Α}$ = $\frac{c_BV_B}{V_{Α}}$ = $\frac{0,05\cdot 0,040}{0,020}$ = $0,10 \frac{mol}{L}$

1.

$pH$ zu Beginn der Titration   $(x=0)$

Die schwache Säure $CH_3COOH$ ist allein vorhanden, ihre Konzentration ist $c_{Α}=0,10 \;M$ Schwache Säure! $pH=\frac{1}{2}pK_a-\frac{1}{2}logc_{Α}$ $=\frac{1}{2}4,75-\frac{1}{2}log0,1$ $pH\;=\;2,88$

2.

$pH$ vor dem Equivalenzpunkt   ($x\;\lt\;40$)

(Der Einfachheit halber betrachten wir die Substanzen zuerst so, als wären sie nicht dissoziiert)

Puffer! $pH$ = $pK_a$ $+$ $log( \frac{n_{CH_3COONa}}{n_{CH_3COOH}})$ = $4,75$ $+$ $log( \frac{0,05\cdot x\cdot10^{-3}}{0,1\cdot20\cdot 10^{-3}-0,05\cdot x\cdot10^{-3}})$ $pH$ $ =$ $ 4,75+log( \frac{0,05\cdot x\cdot}{0,1\cdot20-0,05\cdot x})$

3.

pH am Equivalenzpunkt   ($x\;=\;40$)

$CH_3COOH$ und $NaOH$ haben ganz reagiert, bleibt eine Lösung der schwachen Base $CH_3COO^-$ Schwache Base! $pH$ $ =$ $ 14$ $-$ $\frac{1}{2}pK_B$ $+$ $\frac{1}{2}log (c_{CH_3COONa})$ $ = $ $14$ $-$ $\frac{1}{2}9,25$ $+$ $\frac{1}{2}log (\frac{0,1\cdot20\cdot 10^{-3}}{(20+40)\cdot 10^{-3}})$ $pH = 8,63$

4.

pH nach dem Equivalenzpunkt   ($x\;\gt\;40$)

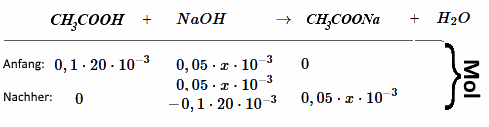
Schwache Base vernachlässigt vor starker Base! Starke Base! $c_{NaOH}$ $= \frac{n_{NaOH}}{(V_{Α}+x)10^{-3}}$ $= \frac{0,05\cdot x\cdot10^{-3}-0,1\cdot20\cdot10^{-3}}{(20+x)10^{-3}}$ $= \frac{0,05\cdot x-2}{20+x}$ $pH$ $ =$ $ 14$ $+$ $log( \frac{0,05\cdot x-2}{20+x})$

→   Hier finden Sie Simulationen von solchen Titrationen